GTOC1: results

The Advanced Concepts Team wishes to thank the 17 teams that participated to this first ACT Global Trajectory Optimisation COmpetition, being aware that they dedicated precious working and free time to make this competition a success.....

"....it was a proof of the scientific level of the participants to be willing to "play" with us testing different methods and techniques with this difficult problem. The ranking reported should by no way be mistaken as absolute: many factors should be considered when evaluating an optimisation method. Its scientific value cannot be judged on the basis of the sole value of the objective function reached in the problem given. Nevertheless this was born as a competition and we had to form a ranking. We hope you enjoyed as much as we did and that the participation to the workshop in February 2006 will be the largest possible." --- Dario Izzo

Other teams returned trajectories that could not be inserted in the above ranking. This was either because the final distance with the asteroid was greater than 0.01 AU or because the format of the submitted file did not comply with the example.txt file provided making it impossible for us to perform a fast preliminary evaluation.

Results

Rank 1: JPL (Team 11)

The trajectory makes use of a planetary sequence EVEEEJSJA. Only the first phase (Earth Venus) is propelled. The overall fuel consumption is of roughly 60kg. The team uses a shape method based on exponential sinusoids to ensure a global search of the solution space. A local optimisation was then used to refine the trajectory based on a NLP solver that is fed with analytical gradients by considering the thrust as a sequence of discrete impulses. The accuracy of this model is high and has been independently verified by forward in time integration.

Rank 2: Deimos Space (Team 17)

The trajectory proposed by this team implies a fly-by sequence EVVEEVVEVEJSJA made of optimsed low-thrust and coast arcs. The overall mass consumption is 82kg which makes the difference with the trajectory found by Team 11 and ranks this trajectory as the second best. They in fact reach the same Jupter-Saturn-Jupiter geometry as the winning trajectory by Jet Propulsion Laboratories, but with a different swing-by sequence at the inner solar system that cost them 20kg of fuel more. This is mainly a consequence of the local optimiser performances. This same fly-by sequence was infact later reoptimised using JPL technique returning a much larger objective function.

Rank 3: GMV (Team 2)

This trajectory implies a EEVEEJSA fly-by sequence with an overall mass consumption of 192kg. The global search technique used is conceptually equal to the one used by the Team 17. It seems that also deep space manouvres have been allowed in this case, when searching for ballistic solutions. The lower objective function value achieved may be due to an incomplete enumerative search of the possible fly-by sequences.

Rank 4: Moscow Aviation Institute & Krunishev Space Center (Team 12)

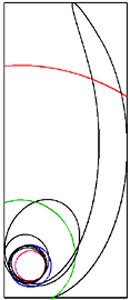
This trajectory makes use of a planetary sequence EVEVEEA without any swing by of the mass giants. The retrograde orbit is obtained by applying a long thrust arc at a high heliocentric distance. The team did not use methods of global optimisation. Some intuitively chosen trajectories were considered. The most successful of them were optimized using the local optimisation methods: Maximum principle; Continuation with respect to boundary conditions; Continuation with respect to gravity parameter; Continuation from the LP (power-limited) problem into CEV (constant ejection velocity) problem.

Rank 5: Politecnico di Torino (Team 4)

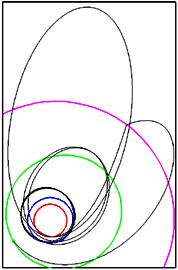
This team has experience mainly with local trajectory optimisation techniques. It selected the planetary sequence by means of experience, intuition and the results of separate optimisation of shorter legs; the coast-thrust structure was found using Pontryagin's maximum principle. An EVVJA sequence was tried and optimised locally converging to the locally optimal solution visualized on the left. The team could not apply their automatic global search strategy as it is currently implemented only for problems with a complex structure but a limited number of flybys.

Rank 6: CNES/CS (Team 7)

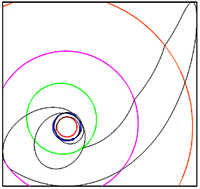
This EEVEEJSJA trajectory is the results of two main steps: a search for a quasi-ballistic multi-swingby first guess solution and the solution of a global optimal control problem. Although the final JSJ sequence was identified and used, the retrograde orbit was obtained by thursting beyond Saturn which increases considerably the propellant consumption.

Rank 7: Glasgow University (Team 13)

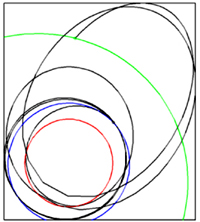
The problem was solved using two method: 1. an evolutionary-branching technique. 2. a set-oriented multilevel subdivision procedure to view iteration for local optimization. The solutions obtained by the two optimization approaches were locally refined via the software DITAN. This solution suffers from the preliminary trade-off between a direct and a retrograde impact. High values of the objective function cannot be achieved in the latter case.

Rank 8: Moscow University (Team 9)

This very interesting trajectory resulted to be the best one within the direct transfers among the trajectories returned before the competition end. Later, at the workshop, an even better direct transfer was presented by the Team of Moscow Aviation Institute and Khrunichev State Research and Production Space Center. A detailed description of the methods used by MSU was not obtained beforehand, but they are contained in the special issue of Acta Astronautica dedicated to the competition results.

Rank 9: Alcatel (Team 14)

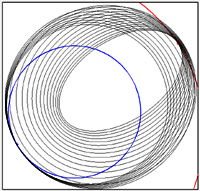
The trajectory is a direct transfer from the Earth to the asteroid TW229. The optimisation method used is mainly Pontryagin maximum principle. The authors did not state how the initial guess was obtained. A trial and error strategy might have been implemented. The final solution is not globally otimal as the trajectory found by Lomonosov's Moscow State University showed.

Rank 10: DLR (Team 1)

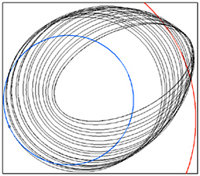
The trajectory is a direct transfer from the Earth to the asteroid TW229. The thrust history is approximated by a neural network whose parameters are then "evolved" with a genetic strategy. No hybrid optimisation is implemented and as a result the final trajectory, though probably in the basin of attraction of a local minima, does not reach precisely the asteroid. As a consequence the final value of J=330000 has been assigned to this trajectory taking into account a penalty. The solution is not globally otimal as the trajectory found by Moscow University shows. The launch and arrival date found by this team are the same as those found by Team 14.

Rank 11: Tsinghua University (Team 8)

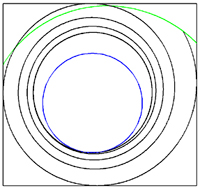
Despite the small value of the objective function achieved the method used by this team is interesting. The trajectory shape is pre imposed and a five dimensional optimisation problem is created. The final randezvous condition is imposed as a penalty function. A genetic strategy is then used to find the best solution. The low value of the objective function is not due to the global optimiser failiure, but to the oversimplification of the trajectory shape.