Nonlinear Propagation of Non-Gaussian Uncertainty
Background
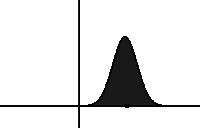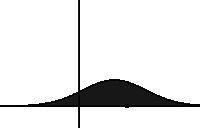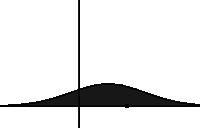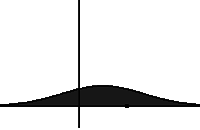
Uncertainty propagation plays a crucial role in various aspects of space trajectory design, including low-Earth orbit determination, satellite tracking, collision avoidance, robust trajectory estimation in deep space missions, and space situational awareness. Approaches to uncertainty propagation vary widely, from attempts to solve the FokkerPlanck equation directly, using approximated or numerical methods, to techniques based on first-order dynamics expansions (e.g., linear covariance propagation), polynomial chaos expansion, and change of variable technique. Other methods leverage statistical properties of Keplerian orbits or apply Gaussian mixture models combined with unscented transformations. One of the most popular methods is via state transition tensors (STT), which leverages the high-order expansion of the flow around a nominal trajectory to map uncertainties at future times [1]. However, methods based on STTs have so far only dealt with initial Gaussian distributions, thereby preventing the treatment of generic probability density functions on both initial conditions and problem parameters.
Project goals
In this project, we studied a novel approach for propagating uncertainties in dynamical systems building on high-order Taylor expansions of the flow and moment-generating functions (MGFs) [2]. Unlike prior methods that focus on Gaussian distributions, our approach leverages the relationship between MGFs and distribution moments to extend high-order uncertainty propagation techniques to non-Gaussian scenarios. This broadens the applicability of these methods to a wider range of problems and uncertainty types. High-order moment computations can be performed one-off and symbolically, reducing the computational burden of the technique to the calculation of Taylor series coefficients around a nominal trajectory, achieved by efficiently integrating the system's variational equations with heyoka. Furthermore, the use of the proposed approach in combination with event transition tensors, allows for accurate propagation of uncertainties at specific events, such as the landing surface of a celestial body, the crossing of a predefined Poincaré section, or the trigger of an arbitrary event during the propagation. Through numerical simulations we studied the effectiveness of the method in various astrodynamics applications, including the unperturbed and perturbed two-body problem, and the circular restricted three-body problem, spacecraft collision avoidance scenarios, as well as controlled landing on irregularly shaped asteroids.
References
[1] Ryan S. Park and Daniel J. Scheeres. "Nonlinear mapping of Gaussian statistics: theory and applications to spacecraft trajectory design." Journal of guidance, Control, and Dynamics 29, no. 6 (2006): 1367-1375.
[2] Giacomo Acciarini, Nicola Baresi, David Lloyd and Dario Izzo. "Nonlinear Propagation of Non-Gaussian Uncertainties". To appear in Journal of Guidance, Control, and Dynamics (2025).