Post-Newtonian orbital mechanics around a black hole in MOdified Gravity
Background
Since the second half of the 20th century, a number of observations of galaxies cannot be reconciled with both of the following assumptions holding at the same time: (i) the observed brightness profile proxies a galaxie's matter distribution; (ii) Newtonian gravity governs the dynamics of these stellar systems. Consequently, attempts to explain this discrepancy cluster into either of the following hypotheses: (a) there is a substantial amount of dark matter in galaxies; (b) the laws of gravity deviate from Newton's laws on galactic scales. (Figure 1)
![Figure 1. Illustration of the observations at the roots of the tree of proposed solutions. (Inspired by Fig. 1 in [1].)](/gsp/ACT/images/projects/pn_mechanics_in_mog_1.png)
While dark matter is the favoured hypothesis by most astronomers today, backed not only by agreements with observations of single galaxies, but also of galaxy clusters and even on cosmological scales, alternatives to Newtonian gravity remain serious contenders. The most prominent such is known as Modified Newtonian Dynamics (MOND), which alters the laws of gravity for low accelerations. Proponents of MOND highlight a more detailed agreement with observations on the scales of single galaxies. Critics of MOND point at its difficulties with an adequate description of the dynamics of galaxy clusters, and to the fact that the hypothesis did not yet yield tests on cosmological scales. Furthermore, MOND recently failed a strong observational test based on the dynamics of stellar binaries with a wide separation. [1], [2]
Another alternative theory of gravity, designed to explain observations without dark matter is known as Modified Gravity (MOG) or scalar-tensor-vector gravity (STVG). MOG explains observations on galactic scales via a stronger than Newtonian gravitational attraction, while attaining the Newtonian laws on small scales via a delicate balance between this stronger attraction and an additional repulsive component of gravity, called fifth force. It has recently been pointed out that MOG would by design pass the wide binary test. Unlike MOND, MOG is also a relativistic theory, allowing not only for cosmological tests, but also for tests in the strong gravity regime, such as around black holes. One parameter of the theory has recently been constrained via infrared observations of a star S2 in orbit around the massive black hole in the centre of the milky way. [3], [4], [5], [6]
Project
We took a closer look at MOG and obtained the following results:
- We derived the post-Newtonian equations of motion of the theory, that is, its law of gravity which dictates the motion in the intermediate regime, where gravity is stronger than in the weak field limit, but at the same time not yet strong enough such that the full theory would be required. An example cases where these equations are valid is the star S2 mentioned above, but our equations also pave the way for potential future high precision tests of MOG in the solar system.
- We assessed the further constrainability of the parameters of the theory and conclude that by continued observations of the star S2, and improvement of the current bound by a factor 1/10 is realistic within about a decade.
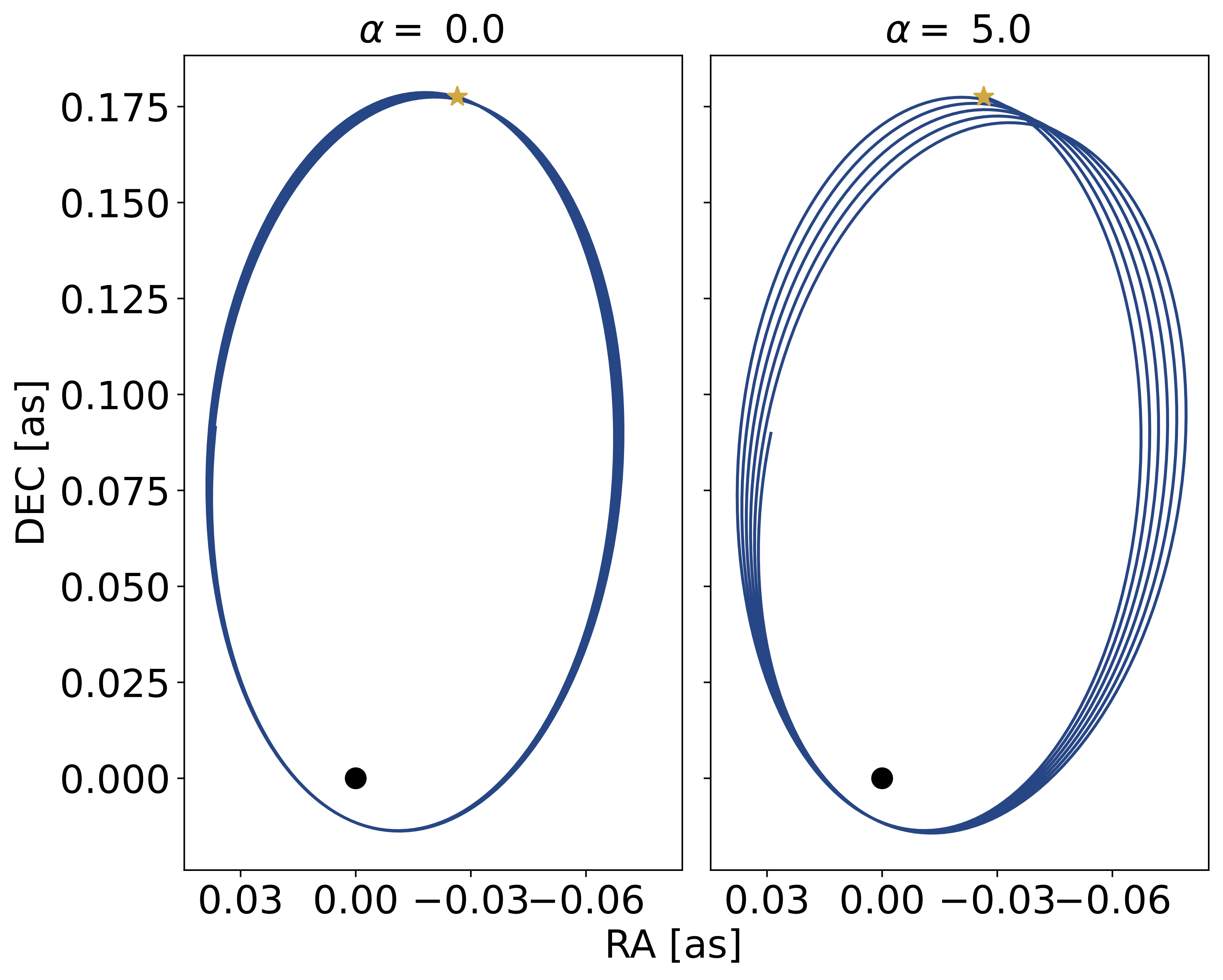
Figure 2. The secular (long-term) effect of MOG onto an orbit is an increased rotation of the orbit (right) compared to that caused by general relativity (left). (To be published in Astronomy & Astrophysics.) - We analyse the orbital mechanics resulting from these equations, and compare it to that arising from general relativity -- the currently accepted theory of gravity. Both theories can yield the same secular (that is, long time-scale) dynamics, though with different parameters. However they are clearly distinguishable via their signature effects on short time-scales. (Figure 2)
- Finally, we detail a mathematical one-to-one correspondence between the dynamics around a black hole in MOG, and the dynamics around an electrically charged black hole in general relativity. While an analogy of these cases has been pointed out before, our result represents a formal equivalence under a certain parameter fine-tuning.
A research article on the above analysis and results is in draft for submission to the peer reviewed journal Astronomy & Astrophysics.
References
[1] Benoît Famaey and Stacy S. McGaugh:
Modified Newtonian Dynamics (MOND): Observational Phenomenology and Relativistic Extensions
Living Rev. Relativity,
15, (2012), 10. http://www.livingreviews.org/lrr-2012-10
[2] Iandranil Banik et al.:
Strong constraints on the gravitational law from Gaia DR3 wide binaries
MNRAS 527, 4573–4615 (2024). https://doi.org/10.1093/mnras/stad3393
[3] John W. Moffat:
Modified gravity (MOG), cosmology and black holes
JCAP 02,(2021), 017. https://doi.org/10.1088/1475-7516/2021/02/017
[4] John W. Moffat:
Black holes in modified gravity (MOG)
Eur. Phys. J. C (2015) 75:175. https://doi.org/10.1140/epjc/s10052-015-3405-x
[5] John W. Moffat:
Wide binaries and modified gravity (MOG)
JCAP, 05, (2024), 079. https://doi.org/10.1088/1475-7516/2024/05/079
[6] Riccardo Della Monica, Ivan de Martino and Mariafelicia de Laurentis:
Orbital precession of the S2 star in Scalar–Tensor–Vector Gravity
MNRAS 510, 4757–4766 (2022). https://doi.org/10.1093/mnras/stab3727